Review for Unit 3 Derivatives Test Ap Calcusus

Defining Average and Instantaneous Rates of Alter at a Point 📍

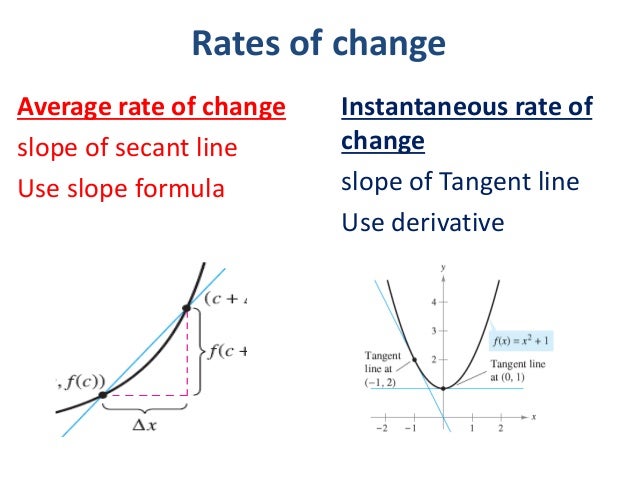
(Photograph courtesy of slideshare.cyberspace)

An of import concept to grasp in this lesson is the divergence between the average rate of change and the instantaneous rate of change. The average rate of change is simply the

gradient of the secant line between ii points

. The instantaneous charge per unit of change is the

slope of the tangent line at any given point

(the derivative).

The rate of change formula (pictured beneath) is the gradient of the secant line between 2 points. "f(b)" represents your y-value to your beginning betoken and "f(a)" represents your y-value to your second betoken, "b" and "a" are the respective 10-values to those coordinates.

• The instantaneous rate of change formula (pictured below) is the slope of the tangent line at a given indicate. Many people are puzzled with f(x+h) but it simply means that whenever you lot see an x in your original equation, y'all insert "x+h." For example, if f(ten) = ten² + 3x - 9 then f(10+h) = (x+h)² + 3(x+h) - nine. The second part of this formula is subtracting f(ten) from f(x+h); nonetheless, a common error is that students forget to distribute the "-" sign to all the terms in the original function. The terminal part is to put everything over h and simplify the entire equation past combining like terms and factoring). If there are any h terms left yous evaluate them equally 0 and so simplify.

Defining the Derivative of a Function and Using Derivative Notation 🤓

The office that gives the instantaneous rate of alter is the derivative, and the derivative is the

slope of the tangent line to the graph at a given bespeak

. The formula for the derivative is the aforementioned as the instantaneous rate of change formula.

The AP examination loves using the notation for the derivative, so don't be scared as they all hateful the same thing; however in certain scenarios in calculus, we may utilize one notation over the other. Here are some of the means nosotros can limited the derivative (pictured below). For now, merely review the notations concerning the starting time derivative. The lesser line is that y' is synonymous with f'(ten) or dy/dx or d/dx f(x)!

Resources:

 (Photo courtesy of didactics.fcps.org)

Connecting Differentiability and Continuity: Determining when Derivatives DO or Do NOT Be ❗

If you need a complete refresher on continuity y'all can sentinel a replay of our stream on Continuity here!

Existence differentiable means that a derivative exists. It is of import to know that being differentiable is being continuous however being continuous does not mean yous are differentiable.

On elevation of beingness continuous in society to be differentiable, the part must take NO corners, cusps, and no vertical tangent lines.

Resources:

Applying the Power Rule 🦸‍♂️🦸‍♀️

Using the definition of the derivative for every single problem you encounter is a time-consuming and it is too open to devil-may-care errors and mistakes. However, one great mathematician decided to bless us with a key rule known as the Power Rule, pictured below.

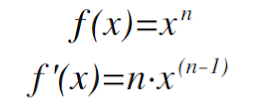
• Your "n" term is the ability your 10-term is existence raised to.

For example, in x² your "n" term would be 2. Additionally, you multiply that "n" value to the term'south coefficient (in x² your coefficient is 1) then subtract your terms exponent by ane (using the power rule the derivative of x² is 2x).

• Remember: the derivative is the gradient of the line tangent to any point on the graph and using the power rule (similar the definition of the derivative) simply gives yous the formula to the gradient of the line tangent. If you would similar to know what that indicate is and then you would have to evaluate using your x-coordinate.

Resource:

Derivative Rules: Abiding, Sum, Difference, and Constant Multiple  ➕➖

These offset prepare of derivative rules are simple simply admittedly crucial to your understanding of calculus.

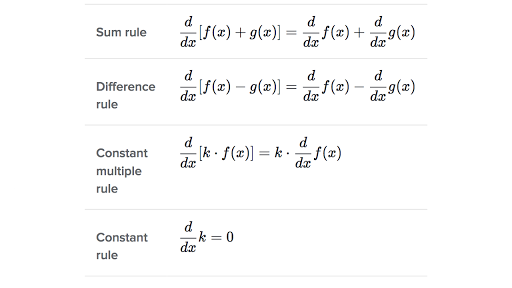
Resources:

(Photograph courtesy of world wide web.khanacademy.org)

• The sum rule states that

  the derivative of a sum of functions is the same as the sum of their derivatives

  .

• Merely like the sum rule, the deviation rule states the derivative of a divergence in functions is the same every bit the difference in their derivatives.

While these two rules may seem confusing, they are actually straightforward. The sum and divergence rules are essentially applications of the power dominion to every term, equally well as combining them (if possible). Here is an case of using both these formulas, pictured beneath:

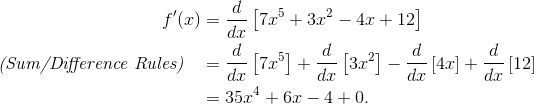
(Photograph courtesy of https://magoosh.com)

• The constant multiple rule states that

  the derivative of a constant times a function is equal to the constant times the derivative

  .

For example, the derivative of 5・ten², (5x²) is equal to 5 times the derivative of 2x.

• The constant rule states that

  the derivative of whatsoever constant is 0

  (for example, the derivative of 5 is 0).

Derivatives of cos, sin, natural exponential functions, and the natural log 🤔

These rules must be committed to memory as they are used throughout the year in calculus.

• The derivative of sin (10) is cos (ten).
• The derivative of cos (x) is -sin (x).

If you would like to find a derivative of a trig function with a constant (such equally 5sin(x)), you would apply the abiding multiple rule to become 5cos(ten).

• It is important to note that the derivative of these functions only work when you are only using "x" in the part. For example, the derivative of sin(3x) is not cos(3x), in social club to get the correct derivative you would demand to use the chain rule. (Practice not worry about the chain rule in this unit as information technology is covered in Unit three of calculus.)

The derivative of e^10 is e^x. No matter how many times y'all have the derivative of this part, the derivative of e^x will remain as eastward^x.

• The derivative of e^x only works when it is raised to only the "x" ability. For example, the derivative e^2x is not e^2x, in social club to get the correct derivative you would need to apply the chain rule.

The derivative of ln(x) is pictured below. If you would want to find the derivative of ln(4x), you would need to apply the chain dominion.

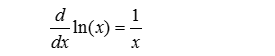
Resource:

The Product Dominion ✖

By now, we know how to add and decrease derivative functions, but what nigh multiplying them? With the production rule we can finally multiply derivatives together. Here is the rule (pictured below)

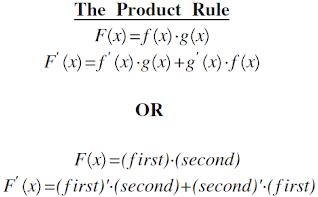
f(x) and one thousand(x) represent two different functions that are being multiplied together. Hither is an example of how to apply this dominion (pictured below).

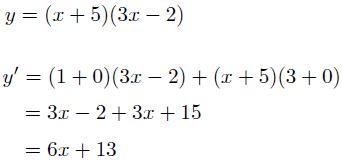
(Photo courtesy of need2knowaboutcalculus.weebly.com)

Let's phone call the first function f(x) and the second function g(x). For our first part, we will take the derivative of the first function and multiply information technology by the original second function. For the 2d part, we and then multiply the original first function and multiply that by the derivative of the 2d function. Finally, we will add together up both parts. If it is helpful to remember the derivative of kickoff times second plus derivative of 2nd times commencement, get for it!

The Quotient Rule ➗

Let'due south now move on to the product dominion'due south partner: the Quotient Dominion! With the quotient dominion, we tin can finally divide derivatives. Hither is what the quotient rule looks like. pictured below:

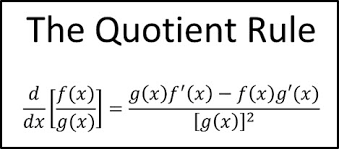
(Photo courtesy of andymath.com)

The quotient rule states that f(ten) is the top function (the dividend) and grand(x) is the bottom role (divisor). The outset role would be to multiply g(10) by the derivative of f(x) and the 2nd office would exist to multiply f(x) by the derivative of g(x). After that, you would decrease the ii parts (don't forget to distribute the negative sign!). Lastly, yous would divide everything past g(10)². If you need another visual, hither is an example (pictured beneath).

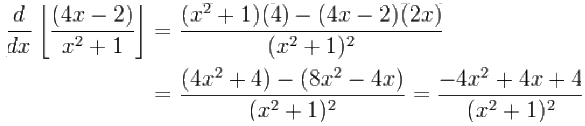
(Photograph courtesy of world wide web.studygeek.org)

Note that (4x-2) is your top function, f(x) and (x²+ane) is your bottom function, thou(x).

Finding the Derivatives of Tangent, Cotangent, Secant, and/or Cosecant Functions 🔎

Here is a helpful chart with the derivatives of the balance of the trigonometric functions also sine and cosine:

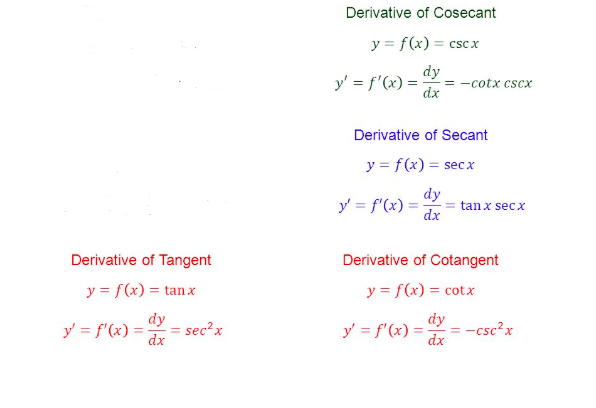
Resource:

(Photo courtesy of slideplayer.com)

Notwithstanding, it is important to accept annotation that AP Calculus mainly focuses on the derivatives of sin, cos, and tan.

Final Note 🎓

Make sure you get the basics down of unit 2 of AP Calculus AB for this unit sets the foundations of calculus, which is essentially the rest of the course. As a result, it is essential for y'all to sympathise these concepts!

heltweepted.blogspot.com

Source: https://fiveable.me/ap-calc/unit-2/unit-2-differentiation-definition-fundamental-properties/blog/gVWfWGHKEn8e8IRNrlSz

Bagikan Artikel ini